Circumference of a Circle

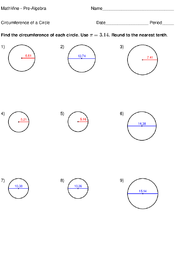
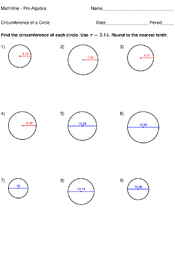
Home > Pre-Algebra > Perimeter > Circumference of a Circle

Circumference of a Circle

Introduction

A circle is a geometric figure which is the set of all points in a plane that are equidistant from a given point called its centre. The circumference of a circle is the distance around all these points. The green circle around the circle below indicates the circumference of this circle.

Terms

Circle - A geometric figure which is the set of all points in a plane that are equidistant from a given point called its centre.

Circumference - The circumference of a circle is the distance around all the points of the circle.

Diameter - A chord that passes through the center of the circle. Equal to twice the radius.

Radius - The distance between of a point that lie on the circle from the center. Equal to half the diameter.

Pi - A constant that describes the ratio between the circumference and diameter of a circle. It is equal to 3.14159..., but typically rounded to 3.14 for calculation.

Lesson

The circumference of a circle can be calculated using the formula $pi * ext{Diameter}$ . So for a circle where the given diameter is 14, the circumference is $pi * 14$ . If we are given the radius of a circle we must first multiply the radius by 2 to get the diameter, and then perform the circumference calculation. So if we are given a circle with a radius of 4, the circumference would be $pi * 4 * 2$ or $pi * 8$

Examples

Circumference of a Circle (Example #1)

Circumference =

\pi

* Diameter

\text{Circumference} = \pi * 14

\text{Circumference} = 43.96

Circumference of a Circle (Example #2)

Circumference = 2 *

\pi

* Radius

\text{Circumference} = 2 * \pi * 8

\text{Circumference} = 50.24

Circumference of a Circle (Example #3)

Circumference =

\pi

* Diameter

\text{Circumference} = \pi * 15.8

\text{Circumference} = 49.61

Circumference of a Circle (Example #4)

Circumference = 2 *

\pi

* Radius

\text{Circumference} = 2 * \pi * 9.9

\text{Circumference} = 62.17