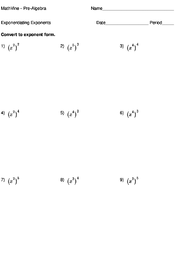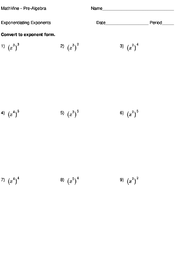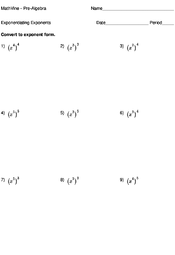Exponentiating Exponents

Home > Pre-Algebra > Exponents > Exponentiating Exponents

Exponentiating Exponents

Introduction

What do we do when the power (exponent) has a power? We simply multiply the exponents together.

Terms

Exponent - The number of times to multiply the base.

Base - The number being multiplied.

Lesson

We could look at it like this: if we are given $(2^3)^2$ , in words that means '2 to the third power, squared'. So if we multiply the exponents together, we get $2^{(3*2)} = 2^6$ or 64 (evaluated).

Here is another example worked out:

$(3^2)^4 = 3^{2*4} = 3^8 = 6561$

Examples

Exponentiating Exponents (Example #1)

\left(x^2\right)^{5}

When you have an exponent expression raised to a power, you have to multiply the two exponents

\left(x^{2}\right)^{5} = x^{(2*5)}

x^10

Exponentiating Exponents (Example #2)

\left(2^4\right)^{3}

When you have an exponent expression raised to a power, you have to multiply the two exponents

\left(2^{4}\right)^{3} = 2^{(4*3)}

2^12

Exponentiating Exponents (Example #3)

\left(2^-4\right)^{3}

When you have an exponent expression raised to a power, you have to multiply the two exponents

\left(2^{-4}\right)^{3} = 2^{(-4*3)}

2^-12

Exponentiating Exponents (Example #4)

\left(8^6\right)^{5}

When you have an exponent expression raised to a power, you have to multiply the two exponents

\left(8^{6}\right)^{5} = 8^{(6*5)}

8^30